Free Vibration Results

 

    

     The variations of bending rigidity, EI, torsion rigidity, GJ, and  bending-torsion coupled stiffness, K, as functions of different ply angles displayed in Figure 4‑9. This plot is particularly important for optimization since a wing composed of fibre-reinforced composite material can be designed for any desired stiffness and corresponding frequency response. A greater flexibility is available with composites which is not necessarily restricted to the plot shown in Figure 4‑9: Plot of Rigidities vs Ply angle for a glass/epoxy composite.Different stacking sequences and ply thickness lead to a much greater domain of possible stiffness properties. Different stacking configurations will be considered in Chapter 5.

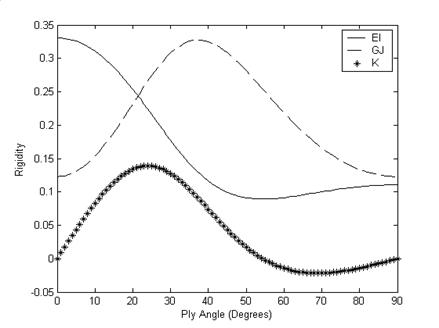
Figure  4‑9: Plot of Rigidities vs Ply angle for a glass/epoxy composite.

     The convergence results for the 1^st four natural frequencies of a uniform glass/epoxy composite beam are presented in Figure 4-10 and the corresponding modes are found in the following Figure 4-10.  It is observed that the DFE and the FEM converge nearly at the same rate for the first three natural frequencies. The DFE converges faster than the FEM for the fourth natural frequency (refer to Figure 4-10). This higher convergence rate can be attributed to the mode behaviour at this natural frequency. The fourth natural frequency is predominately torsion (refer to Figure 4-11 (d)). It has been observed that torsion plays a more important role at higher frequencies and the DFE converges significantly faster than the FEM as the frequency number is increased (Borneman and Hashemi, 2003).

 

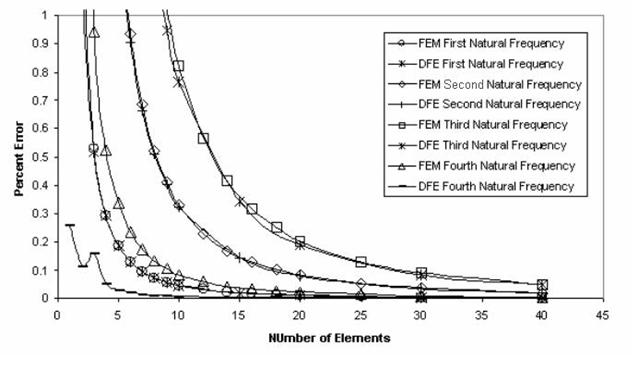
Figure  4‑10: Convergence of DFE and FEM for the first four natural frequencies of a uniform composite wing. Percent Error is relative to the exact values obtained from the DSM  (Banerjee and Williams, 1995).

 

 

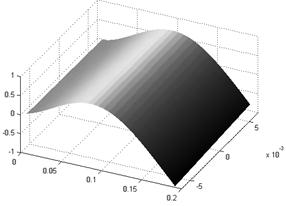
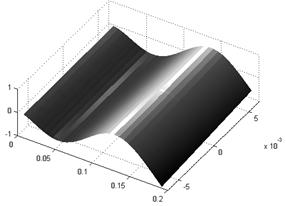
     The two and three dimensional modes of deformation are also plotted in 4-11 (a)-(d) to give a visual representation of the behaviour of the wing when vibrating at the first four natural frequencies. All modes both 2D and 3-D have been normalized to properly distinguish the modes as bending, torsion or bending-torsion. Numerical values of the first five natural frequencies using various methods are presented in Table 4-2.

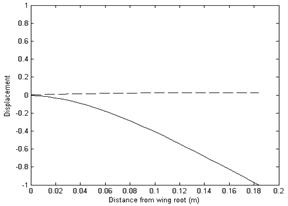
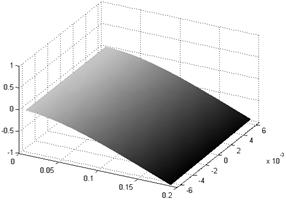
                                    (a)

 

 

 

 

                                    (b)

 

 

                                    (c)       

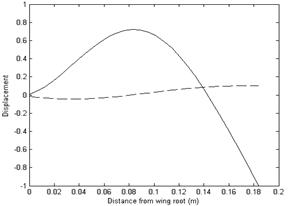
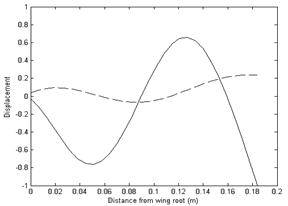
Figure  4‑11: Natural modes of free vibration for a coupled bending-torsion uniform composite beam. (a) First Natural mode; (b) 2^nd Natural mode; (c) 3^rd Natural mode;. Each 2D mode displacement due to torsion is represented by a dashed (--) line and bending is represented by a solid (-) line.

 

                                               

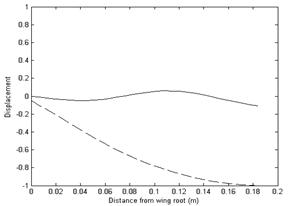
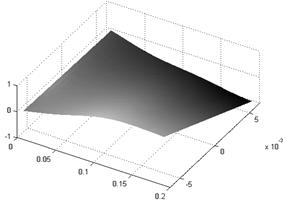
                                    (d)

 

Figure  4‑12: Natural modes of free vibration for a coupled bending-torsion uniform composite beam. (d) 4^th Natural mode. Each 2D mode displacement due to torsion is represented by a dashed (--) line and bending is represented by a solid (-) line.

 

 Table  4‑2: Numerical values of the first five natural frequencies (Hz) using various methods are presented. ‘B’ denotes a predominant bending mode and ‘T’ denotes a predominant torsion mode.

Natural Frequencies of a Uniform Composite Beam 15° lay-up (Hz)
Natural Frequency	FEM 20 Using Elements	DFE Using 20 Elements	DSM 1 Element (Exact)
1st	30.82	30.82 B	30.82
2nd	192.87	192.87 B	192.72
3rd	538.47	538.42 B	537.38
4th	648.87	648.74 T	648.73
5th	1053.87	1053.46 B	1049.73

 

 

     The exact results for the DSM are confirmed by the earlier works published by Banerjee (1998), Banerjee and Williams (1996), Banerjee and Williams (1995) for the first four natural frequencies.

     Figures 4-12 to 4-16 display the variations of natural frequencies for a uniform beam over a range of ply angles. These figures are particularily useful for a quick reference of the ply angle for a desired frequency response. The uniform beam is composed of glass/epoxy composite material with the same dimensions as the first uniform model described in section 4.5.  

 

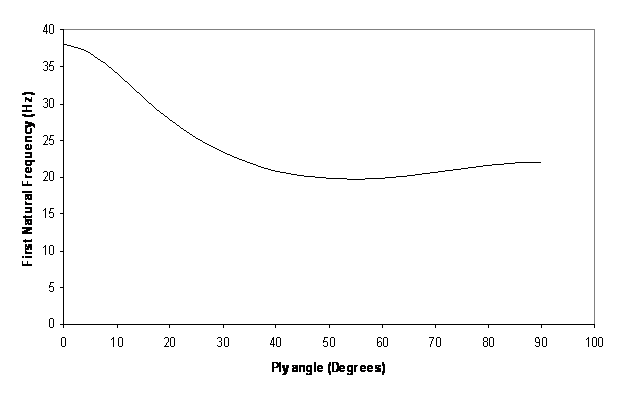
Figure  4‑13: Variations in the first natural frequency for different ply orientations

 

     From Figure 4‑13 it is observed that the first natural frequency starts at its highest point at zero degrees. The natural frequency then decreases and levels out to a constant value at approximately 50 degrees. Similar trends in the second natural frequency are observed where the natural frequency levels at approximately 45 degrees ply orientation in Figure 4‑14.

 

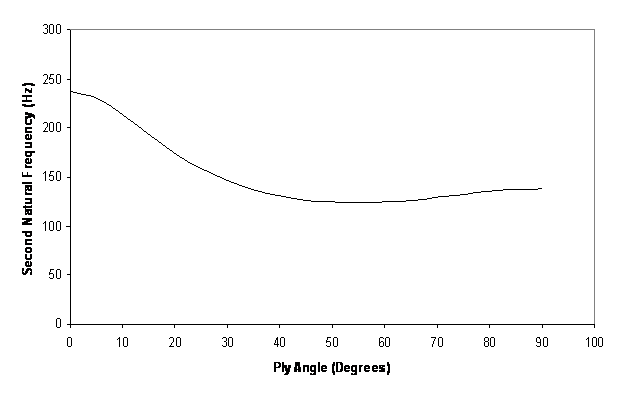
Figure  4‑14: Variations in the second natural frequency for different ply orientations

 

     The third natural frequency (Figure 4‑15) again levels at nearly 50 degrees much like the first mode but an additional increase exists at the initial range from 0-12 degrees. The differences associated with variations in frequency can be attributed to the stronger influence of torsion on the higher modes of materially coupled vibration. This is observed especially in the fourth mode of vibration (Figure 4‑16). The fourth mode displays predominance in torsion (refer to the mode shape from Figure 4-11 (d)). Figure 4‑16 deviates from the original trends found in the first two modes with greater fluctuations in frequency with different ply lay-ups

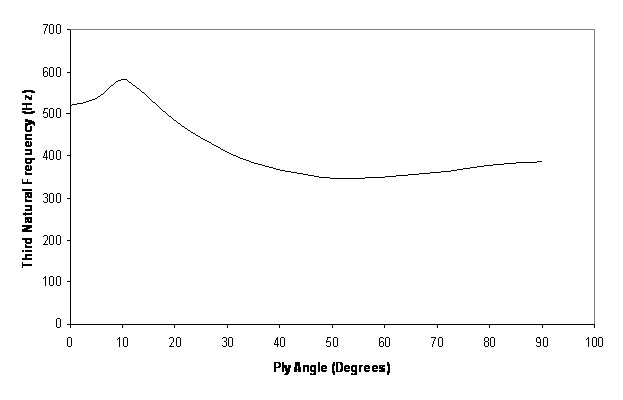
Figure  4‑15: Variations in the third natural frequency for different ply orientations

 

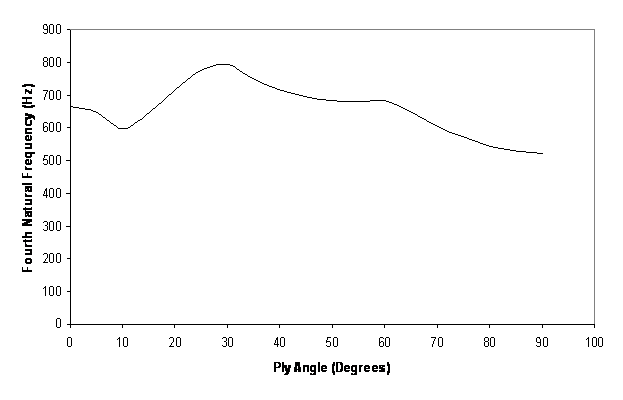
Figure  STYLEREF 1 \s 4‑ SEQ Figure \* ARABIC \s 1 16: Variations in the fourth natural frequency for different ply orientations

 

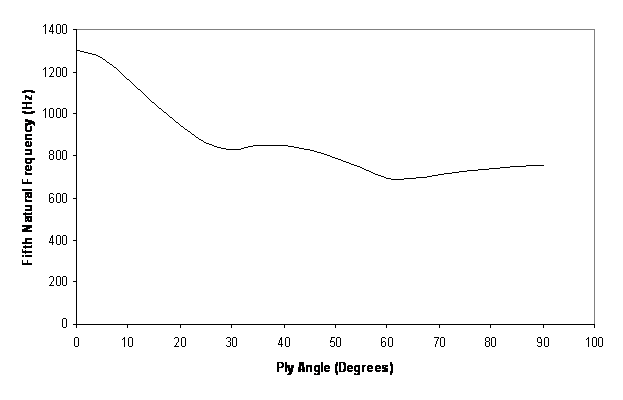
Figure  4‑17: Variations in the fifth natural frequency for different ply orientations

     The fifth natural frequency in Figure 4‑17 returns to the original trend found in the first two predominantly bending modes of vibration (refer to Table 4-2). By extending the results to the fifth mode a correlation is observed between the influence of torsion and the fluctuations in frequency with ply orientation.

 

4.5.2 Numerical example for a step beam

 

More complex geometries such as tapered wings are usually constructed using piecewise uniform steps. The convergence results for a step beam constructed with three steps can be found in Figure 4-17 and Figure 4-18. The beam rigidities at its root (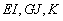) are identical to those of the previous uniform composite beam example and each step has the length of L/3. The second and third steps have the rigidity parameters equal to two-thirds and one-third of those for the root, respectively.

 

 

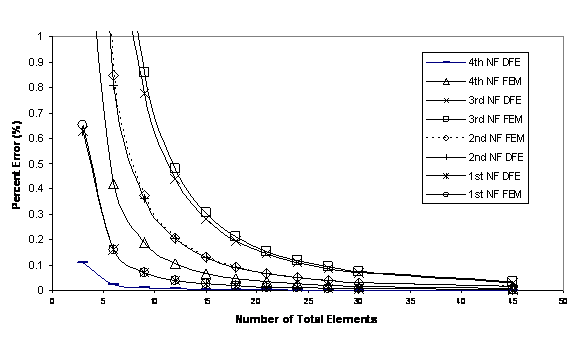
Figure  4‑18: Convergence for a step beam formed from three steps using the FEM and DFE for the first 4 natural frequencies. ‘NF’ represents Natural Frequency.

 

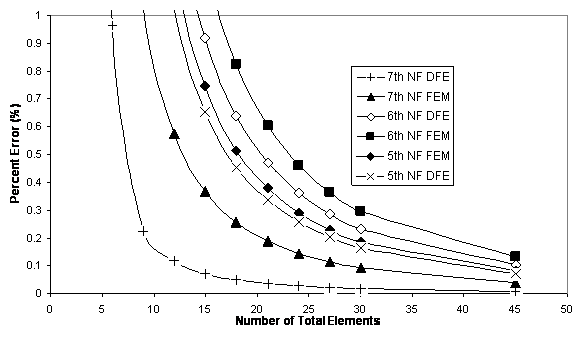
Figure  4‑19: Convergence for a step beam formed from three steps using the FEM and DFE for the 5^th,  6^th, 7^th  natural frequencies.

 

 

     The percent error in Figures 4‑17 and 4-18 is calculated based on the exact values obtained using the DSM method. The first three natural frequencies converged at nearly the same rate as the FEM (see also Borneman and Hashemi, 2003). It is observed from Figures 4-17 and 4‑18 that the DFE converges quicker than the FEM for higher frequencies. If a tapered formulation was used it would include the addition of deviator terms to compensate for the constant parameters assumed over each element. That would increase the convergence rates, and is the factor which distinguishes DFE from DSM method.

 

4.6    Conclusions

 

The DFE displays significantly better convergence than the FEM for higher modes in the cases of the uniform and stepped composite beam. The modes of materially coupled vibration have been classified based on predominance of either bending or torsion and correlations have been drawn based on the higher influence of twist on particular frequencies. Given the fact that the DFE approach is based on a general FEM type formulation the method can be easily extended to more complex element geometries such as tapered elements which will be covered in Chapter 5.